While all students benefit from multiple representations in math, students who have difficulty in math need more experiences with these representations. Those experiences may also need to be more explicit and focused. This post continues the blog series on how to support students who struggle in math by incorporating multiple representations.
Types of Representations
Concrete and semi-concrete representations are powerful ways to make math visible and accessible for students. Creating visual models with concrete or semi-concrete representations help students think through and solve problems successfully because they help students understand the logic of the concepts and procedures.
Sometimes these representations are referred to as CRA: concrete, representation, abstract.
Concrete representations such as base-ten blocks or Cuisenaire Rods allow students to physically model with manipulatives or act out problem scenarios to make concepts visible and less challenging to interpret.
Semi-concretere representations such as strip diagrams, tables, arrays, and number lines are two dimensional representations. They can be used with concrete representations to transition to more abstract representations. For example, students who struggle with addition, subtraction, multiplication, or division may find it useful to represent quantities with tick marks or by drawing dots.
Abstract representations include numbers, equations, operations, relational symbols, and expressions. Far too often math curriculum jumps too quickly to abstract representations or fails to connect the abstract representation to concrete and semi-concrete representations.
You can read more about using math manipulatives here.
Using Representations
It is important to provide students with representations that represent the concept or procedure being taught. Not all representations work for every mathematical concept. When I first started teaching I would try to incorporate all representations, which led to significant frustration.
When possible, try to use representations that are proportional. For example, when teaching place value, the representation for ones should be one-tenth the size of the representation for tens, and the tens should be one-tenth the size of the representation for hundreds. However, when you move to large numbers (see place value chips below) that isn’t always feasible.
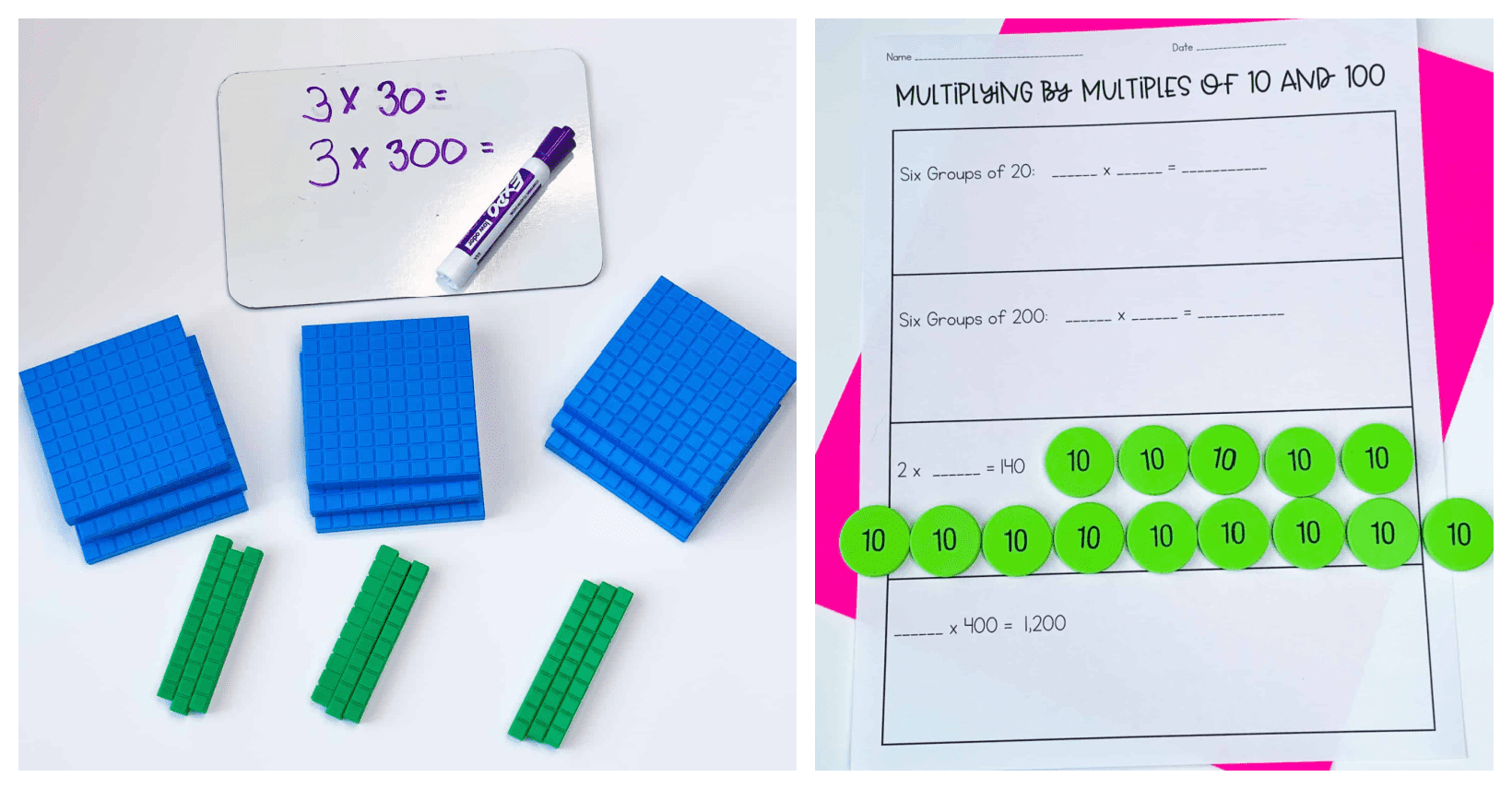
We shouldn’t use representations in isolation. Instead, we should connect concrete and semi-concrete representations to abstract representations throughout our instruction. When we present representations simultaneously students can begin to conceptualize the connection between the representations and the abstract math.
Allowing students to use representations a few times will not be enough for our students who struggle in math. Students need multiple uses to deeply understand concepts. We can fade out concrete and semi-concrete representations as students become accurate with doing the work abstractly. If students do not fully understand the concepts, then fading out is not appropriate.
Number Lines
Number lines are an incredibly important representation. Students who are proficient in math often construct a mental number line as they solve problems. When we consistently uses number lines, students who have difficulty in math gradually develop the ability to visualize number lines.
Number lines can be used to represent whole numbers, fractions, and decimals and build students’ understanding of numerical magnitude. They can also be used to compare numbers and determine their magnitude to help students understand quantity. The understanding of operations can also be reinforced with number lines.
You can see in the pictures below a few examples of how number lines are consistently incorporated in my remediation units. This frequent practice helps students solidify their conceptual understanding.



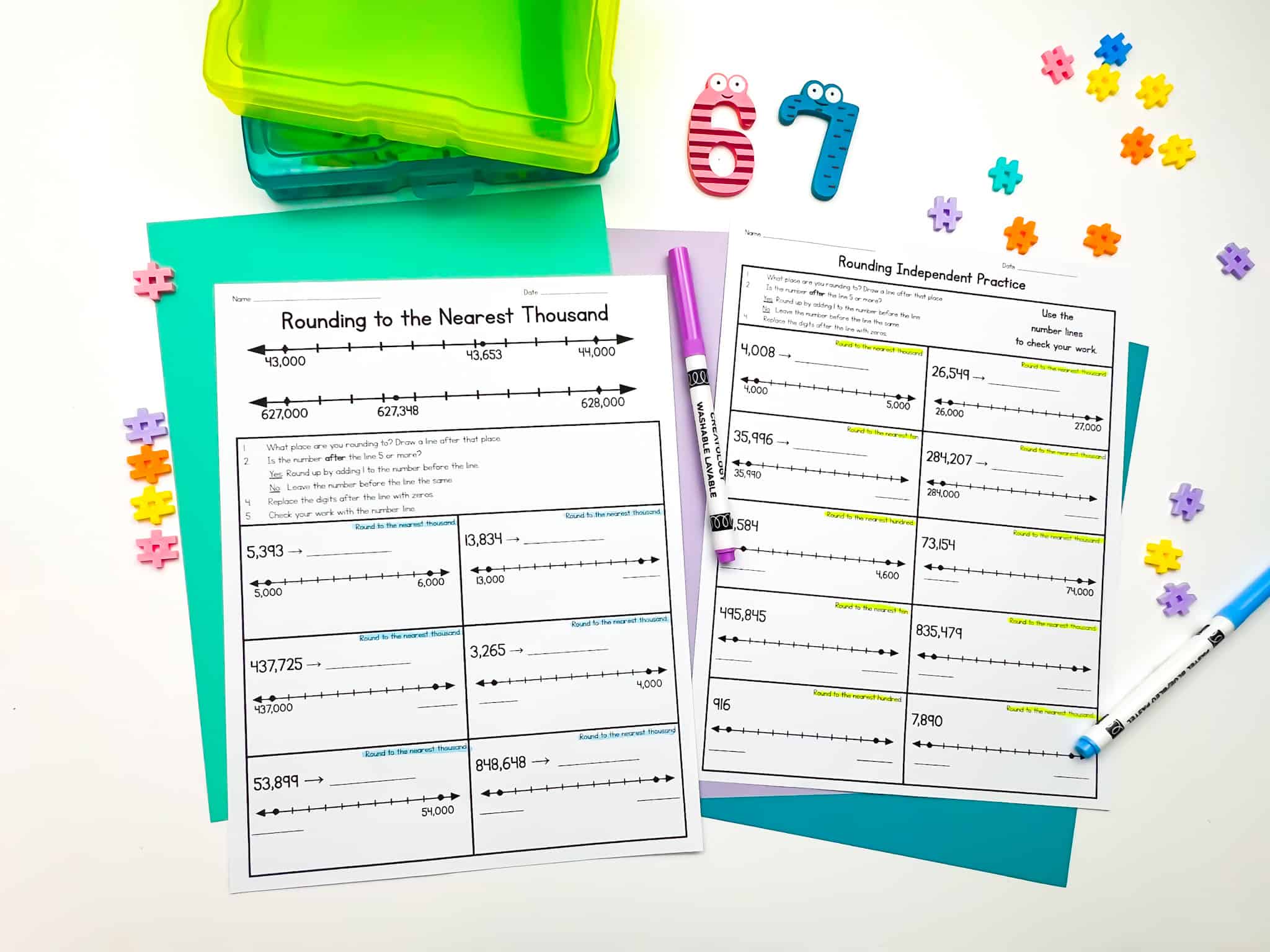
As I create additional remediation units, I will continue to include multiple opportunities for math representations.
Hopefully, you’ve found these strategies useful for all students. You can find more posts for supporting students with difficulty in math using the links below.